Портал:Математика

Темы портала: Деятельность; Культура; География; Здоровье; История; Математика; Природа; Люди; Философия; Религия; Общество; Технологии; Случайный портал

редактировать

Математический портал

П:МАТ
П:МАТЕМАТИКА
П:МАТЕМАТИКА

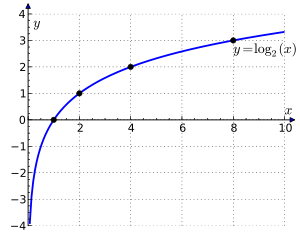
Математика — это наука о представлении и рассуждении об абстрактных объектах (таких как числа , точки , пространства , множества , структуры и игры ). Математика используется во всем мире как важный инструмент во многих областях, включая естественные науки , инженерию , медицину и социальные науки . Прикладная математика , раздел математики, занимающийся применением математических знаний в других областях, вдохновляет и использует новые математические открытия и иногда приводит к развитию совершенно новых математических дисциплин, таких как статистика и теория игр . Математики также занимаются чистой математикой , или математикой ради нее самой, не имея в виду никакого приложения. Не существует четкой границы, разделяющей чистую и прикладную математику, и часто обнаруживаются практические приложения того, что начиналось как чистая математика. ( Полная статья... )

Обновить с новыми вариантами ниже (очистить)

Избранные статьи –@media screen{html.skin-theme-clientpref-night .mw-parser-output div:not(.notheme)>.tmp-color,html.skin-theme-clientpref-night .mw-parser-output p>.tmp-color,html.skin-theme-clientpref-night .mw-parser-output table:not(.notheme) .tmp-color{color:inherit!important}}@media screen and (prefers-color-scheme:dark){html.skin-theme-clientpref-os .mw-parser-output div:not(.notheme)>.tmp-color,html.skin-theme-clientpref-os .mw-parser-output p>.tmp-color,html.skin-theme-clientpref-os .mw-parser-output table:not(.notheme) .tmp-color{color:inherit!important}}загрузить новый пакет

Здесь отображаются избранные статьи , представляющие собой лучший контент английской Википедии.

Image 1

Марка с изображением Чжан Хэна, выпущенная почтой Китая в 1955 году.

Чжан Хэн ( кит .張衡; 78–139 гг. н. э.), ранее романизированный Чан Хэн , был китайским ученым- полиматиком и государственным деятелем, жившим во времена династии Хань . Получив образование в столицах Лоян и Чанъань , он добился успеха как астроном , математик , сейсмолог , инженер-гидравлик , изобретатель , географ , картограф , этнограф , художник , поэт , философ , политик и литературовед.

Чжан Хэн начал свою карьеру в качестве мелкого государственного служащего в Наньяне . В конце концов он стал главным астрономом, префектом майоров официальных экипажей, а затем дворцовым служителем при императорском дворе. Его бескомпромиссная позиция по историческим и календарным вопросам привела к тому, что он стал противоречивой фигурой, что помешало ему подняться до статуса великого историка. Его политическое соперничество с дворцовыми евнухами во время правления императора Шуня (годы правления 125–144) привело к его решению уйти из центрального двора, чтобы служить администратором королевства Хэцзянь в современном Хэбэе . Чжан вернулся домой в Наньян на короткое время, прежде чем был отозван на службу в столицу еще раз в 138 году. Он умер там год спустя, в 139 году. ( Полная статья... )
Image 2
Figure 1: A solution (in purple) to Apollonius's problem. The given circles are shown in black.

In Euclidean plane geometry, Apollonius's problem is to construct circles that are tangent to three given circles in a plane (Figure 1). Apollonius of Perga (c. 262 BC – c. 190 BC) posed and solved this famous problem in his work Ἐπαφαί (Epaphaí, "Tangencies"); this work has been lost, but a 4th-century AD report of his results by Pappus of Alexandria has survived. Three given circles generically have eight different circles that are tangent to them (Figure 2), a pair of solutions for each way to divide the three given circles in two subsets (there are 4 ways to divide a set of cardinality 3 in 2 parts).

In the 16th century, Adriaan van Roomen solved the problem using intersecting hyperbolas, but this solution does not use only straightedge and compass constructions. François Viète found such a solution by exploiting limiting cases: any of the three given circles can be shrunk to zero radius (a point) or expanded to infinite radius (a line). Viète's approach, which uses simpler limiting cases to solve more complicated ones, is considered a plausible reconstruction of Apollonius' method. The method of van Roomen was simplified by Isaac Newton, who showed that Apollonius' problem is equivalent to finding a position from the differences of its distances to three known points. This has applications in navigation and positioning systems such as LORAN. (Full article...)
Image 3
Euclid's method for finding the greatest common divisor (GCD) of two starting lengths BA and DC, both defined to be multiples of a common "unit" length. The length DC being shorter, it is used to "measure" BA, but only once because the remainder EA is less than DC. EA now measures (twice) the shorter length DC, with remainder FC shorter than EA. Then FC measures (three times) length EA. Because there is no remainder, the process ends with FC being the GCD. On the right Nicomachus's example with numbers 49 and 21 resulting in their GCD of 7 (derived from Heath 1908:300).

In mathematics, the Euclidean algorithm, or Euclid's algorithm, is an efficient method for computing the greatest common divisor (GCD) of two integers (numbers), the largest number that divides them both without a remainder. It is named after the ancient Greek mathematician Euclid, who first described it in his Elements (c. 300 BC).
It is an example of an algorithm, a step-by-step procedure for performing a calculation according to well-defined rules,
and is one of the oldest algorithms in common use. It can be used to reduce fractions to their simplest form, and is a part of many other number-theoretic and cryptographic calculations.

The Euclidean algorithm is based on the principle that the greatest common divisor of two numbers does not change if the larger number is replaced by its difference with the smaller number. For example, 21 is the GCD of 252 and 105 (as $252 = 21 \times 12$ and $105 = 21 \times 5)$ , and the same number 21 is also the GCD of 105 and $252 - 105 = 147$ . Since this replacement reduces the larger of the two numbers, repeating this process gives successively smaller pairs of numbers until the two numbers become equal. When that occurs, that number is the GCD of the original two numbers. By reversing the steps or using the extended Euclidean algorithm, the GCD can be expressed as a linear combination of the two original numbers, that is the sum of the two numbers, each multiplied by an integer (for example, $21 = 5 \times 105 + (-2) \times 252$ ). The fact that the GCD can always be expressed in this way is known as Bézout's identity. (Full article...)
Image 4

Richard Phillips Feynman (/ˈfaɪnmən/; May 11, 1918 – February 15, 1988) was an American theoretical physicist. He is known for the work he did in the path integral formulation of quantum mechanics, the theory of quantum electrodynamics, the physics of the superfluidity of supercooled liquid helium, and in particle physics, for which he proposed the parton model. For his contributions to the development of quantum electrodynamics, Feynman received the Nobel Prize in Physics in 1965 jointly with Julian Schwinger and Shin'ichirō Tomonaga.

Feynman developed a widely used pictorial representation scheme for the mathematical expressions describing the behavior of subatomic particles, which later became known as Feynman diagrams. During his lifetime, Feynman became one of the best-known scientists in the world. In a 1999 poll of 130 leading physicists worldwide by the British journal Physics World, he was ranked the seventh-greatest physicist of all time. (Full article...)
Image 5

Bust of Shen at the Beijing Ancient Observatory

Shen Kuo (Chinese: 沈括; 1031–1095) or Shen Gua, courtesy name Cunzhong (存中) and pseudonym Mengqi (now usually given as Mengxi) Weng (夢溪翁), was a Chinese polymath, scientist, and statesman of the Song dynasty (960–1279). Shen was a master in many fields of study including mathematics, optics, and horology. In his career as a civil servant, he became a finance minister, governmental state inspector, head official for the Bureau of Astronomy in the Song court, Assistant Minister of Imperial Hospitality, and also served as an academic chancellor. At court his political allegiance was to the Reformist faction known as the New Policies Group, headed by Chancellor Wang Anshi (1021–1085).

In his Dream Pool Essays or Dream Torrent Essays (夢溪筆談; Mengxi Bitan) of 1088, Shen was the first to describe the magnetic needle compass, which would be used for navigation (first described in Europe by Alexander Neckam in 1187). Shen discovered the concept of true north in terms of magnetic declination towards the north pole, with experimentation of suspended magnetic needles and "the improved meridian determined by Shen's [astronomical] measurement of the distance between the pole star and true north". This was the decisive step in human history to make compasses more useful for navigation, and may have been a concept unknown in Europe for another four hundred years (evidence of German sundials made circa 1450 show markings similar to Chinese geomancers' compasses in regard to declination). (Full article...)
Image 6
The weighing pans of this balance scale contain zero objects, divided into two equal groups.

In mathematics, zero is an even number. In other words, its parity—the quality of an integer being even or odd—is even. This can be easily verified based on the definition of "even": zero is an integer multiple of 2, specifically 0 × 2. As a result, zero shares all the properties that characterize even numbers: for example, 0 is neighbored on both sides by odd numbers, any decimal integer has the same parity as its last digit—so, since 10 is even, 0 will be even, and if $y$ is even then $y + x$ has the same parity as $x$ —indeed, $0 + x$ and $x$ always have the same parity.

Zero also fits into the patterns formed by other even numbers. The parity rules of arithmetic, such as even − even = even, require 0 to be even. Zero is the additive identity element of the group of even integers, and it is the starting case from which other even natural numbers are recursively defined. Applications of this recursion from graph theory to computational geometry rely on zero being even. Not only is 0 divisible by 2, it is divisible by every power of 2, which is relevant to the binary numeral system used by computers. In this sense, 0 is the "most even" number of all. (Full article...)
Image 7

Josiah Willard Gibbs (/ɡɪbz/; February 11, 1839 – April 28, 1903) was an American scientist who made significant theoretical contributions to physics, chemistry, and mathematics. His work on the applications of thermodynamics was instrumental in transforming physical chemistry into a rigorous deductive science. Together with James Clerk Maxwell and Ludwig Boltzmann, he created statistical mechanics (a term that he coined), explaining the laws of thermodynamics as consequences of the statistical properties of ensembles of the possible states of a physical system composed of many particles. Gibbs also worked on the application of Maxwell's equations to problems in physical optics. As a mathematician, he created modern vector calculus (independently of the British scientist Oliver Heaviside, who carried out similar work during the same period) and described the Gibbs phenomenon in the theory of Fourier analysis.

In 1863, Yale University awarded Gibbs the first American doctorate in engineering. After a three-year sojourn in Europe, Gibbs spent the rest of his career at Yale, where he was a professor of mathematical physics from 1871 until his death in 1903. Working in relative isolation, he became the earliest theoretical scientist in the United States to earn an international reputation and was praised by Albert Einstein as "the greatest mind in American history". In 1901, Gibbs received what was then considered the highest honor awarded by the international scientific community, the Copley Medal of the Royal Society of London, "for his contributions to mathematical physics". (Full article...)
Image 8

Émile Michel Hyacinthe Lemoine (French: [emil ləmwan]; 22 November 1840 – 21 February 1912) was a French civil engineer and a mathematician, a geometer in particular. He was educated at a variety of institutions, including the Prytanée National Militaire and, most notably, the École Polytechnique. Lemoine taught as a private tutor for a short period after his graduation from the latter school.

Lemoine is best known for his proof of the existence of the Lemoine point (or the symmedian point) of a triangle. Other mathematical work includes a system he called Géométrographie and a method which related algebraic expressions to geometric objects. He has been called a co-founder of modern triangle geometry, as many of its characteristics are present in his work. (Full article...)
Image 9
The regular triangular tiling of the plane, whose symmetries are described by the affine symmetric group $S̃ 3$

The affine symmetric groups are a family of mathematical structures that describe the symmetries of the number line and the regular triangular tiling of the plane, as well as related higher-dimensional objects. In addition to this geometric description, the affine symmetric groups may be defined in other ways: as collections of permutations (rearrangements) of the integers ( $..., -2, -1, 0, 1, 2, ...$ ) that are periodic in a certain sense, or in purely algebraic terms as a group with certain generators and relations. They are studied in combinatorics and representation theory.

A finite symmetric group consists of all permutations of a finite set. Each affine symmetric group is an infinite extension of a finite symmetric group. Many important combinatorial properties of the finite symmetric groups can be extended to the corresponding affine symmetric groups. Permutation statistics such as descents and inversions can be defined in the affine case. As in the finite case, the natural combinatorial definitions for these statistics also have a geometric interpretation. (Full article...)
Image 10
General relativity, also known as the general theory of relativity, and as Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. General relativity generalizes special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time, or four-dimensional spacetime. In particular, the curvature of spacetime is directly related to the energy and momentum of whatever present matter and radiation. The relation is specified by the Einstein field equations, a system of second-order partial differential equations.

Newton's law of universal gravitation, which describes classical gravity, can be seen as a prediction of general relativity for the almost flat spacetime geometry around stationary mass distributions. Some predictions of general relativity, however, are beyond Newton's law of universal gravitation in classical physics. These predictions concern the passage of time, the geometry of space, the motion of bodies in free fall, and the propagation of light, and include gravitational time dilation, gravitational lensing, the gravitational redshift of light, the Shapiro time delay and singularities/black holes. So far, all tests of general relativity have been shown to be in agreement with the theory. The time-dependent solutions of general relativity enable us to talk about the history of the universe and have provided the modern framework for cosmology, thus leading to the discovery of the Big Bang and cosmic microwave background radiation. Despite the introduction of a number of alternative theories, general relativity continues to be the simplest theory consistent with experimental data. (Full article...)
Image 11

Kaczynski after his arrest in 1996

Theodore John Kaczynski (/kəˈzɪnski/ ^ⓘ kə-ZIN-skee; May 22, 1942 – June 10, 2023), also known as the Unabomber (/ˈjuːnəbɒmər/ ^ⓘ YOO-nə-bom-ər), was an American mathematician and domestic terrorist. He was a mathematics prodigy, but abandoned his academic career in 1969 to pursue a reclusive primitive lifestyle.

Kaczynski murdered three people and injured 23 others between 1978 and 1995 in a nationwide mail bombing campaign against people he believed to be advancing modern technology and the destruction of the natural environment. He authored Industrial Society and Its Future, a 35,000-word manifesto and social critique which opposes all forms of technology, rejecting leftism, and advocating a nature-centered form of anarchism. (Full article...)
Image 12

The title page of a 1634 version of Hues' Tractatus de globis in the collection of the Biblioteca Nacional de Portugal

Robert Hues (1553 – 24 May 1632) was an English mathematician and geographer. He attended St. Mary Hall at Oxford, and graduated in 1578. Hues became interested in geography and mathematics, and studied navigation at a school set up by Walter Raleigh. During a trip to Newfoundland, he made observations which caused him to doubt the accepted published values for variations of the compass. Between 1586 and 1588, Hues travelled with Thomas Cavendish on a circumnavigation of the globe, performing astronomical observations and taking the latitudes of places they visited. Beginning in August 1591, Hues and Cavendish again set out on another circumnavigation of the globe. During the voyage, Hues made astronomical observations in the South Atlantic, and continued his observations of the variation of the compass at various latitudes and at the Equator. Cavendish died on the journey in 1592, and Hues returned to England the following year.

In 1594, Hues published his discoveries in the Latin work Tractatus de globis et eorum usu (Treatise on Globes and Their Use) which was written to explain the use of the terrestrial and celestial globes that had been made and published by Emery Molyneux in late 1592 or early 1593, and to encourage English sailors to use practical astronomical navigation. Hues' work subsequently went into at least 12 other printings in Dutch, English, French and Latin. (Full article...)
Image 13

Title page of the first edition of Wright's Certaine Errors in Navigation (1599)

Edward Wright (baptised 8 October 1561; died November 1615) was an English mathematician and cartographer noted for his book Certaine Errors in Navigation (1599; 2nd ed., 1610), which for the first time explained the mathematical basis of the Mercator projection by building on the works of Pedro Nunes, and set out a reference table giving the linear scale multiplication factor as a function of latitude, calculated for each minute of arc up to a latitude of 75°. This was in fact a table of values of the integral of the secant function, and was the essential step needed to make practical both the making and the navigational use of Mercator charts.

Wright was born at Garveston in Norfolk and educated at Gonville and Caius College, Cambridge, where he became a fellow from 1587 to 1596. In 1589 the college granted him leave after Elizabeth I requested that he carry out navigational studies with a raiding expedition organised by the Earl of Cumberland to the Azores to capture Spanish galleons. The expedition's route was the subject of the first map to be prepared according to Wright's projection, which was published in Certaine Errors in 1599. The same year, Wright created and published the first world map produced in England and the first to use the Mercator projection since Gerardus Mercator's original 1569 map. (Full article...)
Image 14

Noether c. 1900–1910

Amalie Emmy Noether (US: /ˈnʌtər/, UK: /ˈnɜːtə/; German: [ˈnøːtɐ]; 23 March 1882 – 14 April 1935) was a German mathematician who made many important contributions to abstract algebra. She proved Noether's first and second theorems, which are fundamental in mathematical physics. She was described by Pavel Alexandrov, Albert Einstein, Jean Dieudonné, Hermann Weyl and Norbert Wiener as the most important woman in the history of mathematics. As one of the leading mathematicians of her time, she developed theories of rings, fields, and algebras. In physics, Noether's theorem explains the connection between symmetry and conservation laws.

Noether was born to a Jewish family in the Franconian town of Erlangen; her father was the mathematician Max Noether. She originally planned to teach French and English after passing the required examinations but instead studied mathematics at the University of Erlangen, where her father lectured. After completing her doctorate in 1907 under the supervision of Paul Gordan, she worked at the Mathematical Institute of Erlangen without pay for seven years. At the time, women were largely excluded from academic positions. In 1915, she was invited by David Hilbert and Felix Klein to join the mathematics department at the University of Göttingen, a world-renowned center of mathematical research. The philosophical faculty objected, however, and she spent four years lecturing under Hilbert's name. Her habilitation was approved in 1919, allowing her to obtain the rank of Privatdozent. (Full article...)
Image 15

Cantor, c. 1910

Georg Ferdinand Ludwig Philipp Cantor (/ˈkæntɔːr/ KAN-tor; German: [ˈɡeːɔʁk ˈfɛʁdinant ˈluːtvɪç ˈfiːlɪp ˈkantoːɐ̯]; 3 March [O.S. 19 February] 1845 – 6 January 1918) was a mathematician who played a pivotal role in the creation of set theory, which has become a fundamental theory in mathematics. Cantor established the importance of one-to-one correspondence between the members of two sets, defined infinite and well-ordered sets, and proved that the real numbers are more numerous than the natural numbers. Cantor's method of proof of this theorem implies the existence of an infinity of infinities. He defined the cardinal and ordinal numbers and their arithmetic. Cantor's work is of great philosophical interest, a fact he was well aware of.

Originally, Cantor's theory of transfinite numbers was regarded as counter-intuitive – even shocking. This caused it to encounter resistance from mathematical contemporaries such as Leopold Kronecker and Henri Poincaré and later from Hermann Weyl and L. E. J. Brouwer, whilst Ludwig Wittgenstein raised philosophical objections; see Controversy over Cantor's theory. Cantor, a devout Lutheran Christian, believed the theory had been communicated to him by God. Some Christian theologians (particularly neo-Scholastics) saw Cantor's work as a challenge to the uniqueness of the absolute infinity in the nature of God – on one occasion equating the theory of transfinite numbers with pantheism – a proposition that Cantor vigorously rejected. Not all theologians were against Cantor's theory; prominent neo-scholastic philosopher Constantin Gutberlet was in favor of it and Cardinal Johann Baptist Franzelin accepted it as a valid theory (after Cantor made some important clarifications). (Full article...)

редактировать

Выбранное изображение –показать еще

ASCII-арт-изображение множества Мандельброта с низким разрешением

Множество Мандельброта

Кредит: Эльфаба

Это современная репродукция первого опубликованного изображения множества Мандельброта , которое появилось в 1978 году в технической статье о группах Клейна Роберта В. Брукса и Питера Мательски. Множество Мандельброта состоит из точек

c

на комплексной плоскости , которые генерируют ограниченную последовательность значений, когда рекурсивное соотношение

z n +1 = z n 2 + c

многократно применяется, начиная с

z 0 = 0.

Граница множества представляет собой очень сложный фрактал , раскрывающий все более мелкие детали при возрастающем увеличении. Граница также включает в себя меньшие почти копии общей формы, явление, известное как квазисамоподобие . Изображение ASCII-арта , видимое на этом изображении, лишь намекает на сложность границы множества. Достижения в области вычислительной мощности и компьютерной графики в 1980-х годах привели к публикации цветных изображений множества с высоким разрешением (на которых цвета точек вне множества отражают, насколько быстро расходятся соответствующие последовательности комплексных чисел), и сделали множество Мандельброта широко известным широкой публике. Названное математиками Адриеном Дуади и Джоном Х. Хаббардом в честь Бенуа Мандельброта , одного из первых математиков, подробно изучивших множество, множество Мандельброта тесно связано с множеством Жюлиа , которое изучал Гастон Жюлиа с 1910-х годов.

Хорошие статьи –загрузить новую партию

Это хорошие статьи , которые соответствуют основным высоким редакционным стандартам.

Image 1
Мозаика Пенроуза с ромбами, обладающими пятикратной симметрией

Плитка Пенроуза является примером апериодической плитки . Здесь плитка представляет собой покрытие плоскости неперекрывающимися многоугольниками или другими фигурами, и плитка является апериодической , если она не содержит произвольно больших периодических областей или участков. Однако, несмотря на отсутствие трансляционной симметрии , плитки Пенроуза могут иметь как симметрию отражения , так и пятикратную вращательную симметрию . Плитки Пенроуза названы в честь математика и физика Роджера Пенроуза , который исследовал их в 1970-х годах.

Существует несколько вариантов плиток Пенроуза с различными формами плиток. Первоначальная форма плитки Пенроуза использовала плитки четырех различных форм, но позже она была сокращена только до двух форм: либо два различных ромба , либо два различных четырехугольника, называемых воздушными змеями и дротиками. Плитки Пенроуза получаются путем ограничения способов, которыми эти фигуры могут совмещаться таким образом, чтобы избежать периодической плитки. Это можно сделать несколькими способами, включая правила сопоставления, подстановку мозаики или правила конечного подразделения , схемы разрезания и проекции, а также покрытия. Даже ограниченная таким образом, каждая вариация дает бесконечно много различных мозаик Пенроуза. ( Полная статья... )
Image 2
Graph of $log 2 x$ as a function of a positive real number $x$

In mathematics, the binary logarithm ( $log 2 n$ ) is the power to which the number $2$ must be raised to obtain the value $n$ . That is, for any real number $x$ ,
: $x=\log _{2}n\quad \Longleftrightarrow \quad 2^{x}=n.$
For example, the binary logarithm of $1$ is $0$ , the binary logarithm of $2$ is $1$ , the binary logarithm of $4$ is $2$ , and the binary logarithm of $32$ is $5$ .

The binary logarithm is the logarithm to the base $2$ and is the inverse function of the power of two function. As well as $log 2$ , an alternative notation for the binary logarithm is $lb$ (the notation preferred by ISO 31-11 and ISO 80000-2). (Full article...)
Image 3
A well-covered graph, the intersection graph of the nine diagonals of a hexagon. The three red vertices form one of its 14 equal-sized maximal independent sets, and the six blue vertices form the complementary minimal vertex cover.

In graph theory, a well-covered graph is an undirected graph in which the minimal vertex covers all have the same size. Here, a vertex cover is a set of vertices that touches all edges, and it is minimal if removing any vertex from it would leave some edge uncovered. Equivalently, well-covered graphs are the graphs in which all maximal independent sets have equal size. Well-covered graphs were defined and first studied by Michael D. Plummer in 1970.

The well-covered graphs include all complete graphs, balanced complete bipartite graphs, and the rook's graphs whose vertices represent squares of a chessboard and edges represent moves of a chess rook. Known characterizations of the well-covered cubic graphs, well-covered claw-free graphs, and well-covered graphs of high girth allow these graphs to be recognized in polynomial time, but testing whether other kinds of graph are well-covered is a coNP-complete problem. (Full article...)
Image 4
Richard Wesley Hamming (February 11, 1915 – January 7, 1998) was an American mathematician whose work had many implications for computer engineering and telecommunications. His contributions include the Hamming code (which makes use of a Hamming matrix), the Hamming window, Hamming numbers, sphere-packing (or Hamming bound), Hamming graph concepts, and the Hamming distance.

Born in Chicago, Hamming attended University of Chicago, University of Nebraska and the University of Illinois at Urbana–Champaign, where he wrote his doctoral thesis in mathematics under the supervision of Waldemar Trjitzinsky (1901–1973). In April 1945, he joined the Manhattan Project at the Los Alamos Laboratory, where he programmed the IBM calculating machines that computed the solution to equations provided by the project's physicists. He left to join the Bell Telephone Laboratories in 1946. Over the next fifteen years, he was involved in nearly all of the laboratories' most prominent achievements. For his work, he received the Turing Award in 1968, being its third recipient. (Full article...)
Image 5
16 polygonalizations of a set of six points

In computational geometry, a polygonalization of a finite set of points in the Euclidean plane is a simple polygon with the given points as its vertices. A polygonalization may also be called a polygonization, simple polygonalization, Hamiltonian polygon, non-crossing Hamiltonian cycle, or crossing-free straight-edge spanning cycle.

Every point set that does not lie on a single line has at least one polygonalization, which can be found in polynomial time. For points in convex position, there is only one, but for some other point sets there can be exponentially many. Finding an optimal polygonalization under several natural optimization criteria is a hard problem, including as a special case the travelling salesman problem. The complexity of counting all polygonalizations remains unknown. (Full article...)
Image 6

Escher in 1971

Maurits Cornelis Escher (Dutch pronunciation: [ˈmʌurɪts kɔrˈneːlɪs ˈɛɕər]; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made woodcuts, lithographs, and mezzotints, many of which were inspired by mathematics.
Despite wide popular interest, for most of his life Escher was neglected in the art world, even in his native Netherlands. He was 70 before a retrospective exhibition was held. In the late twentieth century, he became more widely appreciated, and in the twenty-first century he has been celebrated in exhibitions around the world.

His work features mathematical objects and operations including impossible objects, explorations of infinity, reflection, symmetry, perspective, truncated and stellated polyhedra, hyperbolic geometry, and tessellations. Although Escher believed he had no mathematical ability, he interacted with the mathematicians George Pólya, Roger Penrose, and Donald Coxeter, and the crystallographer Friedrich Haag, and conducted his own research into tessellation. (Full article...)
Image 7

Equilateral form of the Cairo tiling

In geometry, a Cairo pentagonal tiling is a tessellation of the Euclidean plane by congruent convex pentagons, formed by overlaying two tessellations of the plane by hexagons and named for its use as a paving design in Cairo. It is also called MacMahon's net after Percy Alexander MacMahon, who depicted it in his 1921 publication New Mathematical Pastimes. John Horton Conway called it a 4-fold pentille.

Infinitely many different pentagons can form this pattern, belonging to two of the 15 families of convex pentagons that can tile the plane. Their tilings have varying symmetries; all are face-symmetric. One particular form of the tiling, dual to the snub square tiling, has tiles with the minimum possible perimeter among all pentagonal tilings. Another, overlaying two flattened tilings by regular hexagons, is the form used in Cairo and has the property that every edge is collinear with infinitely many other edges. (Full article...)
Image 8
The brute force algorithm finds a 4-clique in this 7-vertex graph (the complement of the 7-vertex path graph) by systematically checking all C(7,4) = 35 4-vertex subgraphs for completeness.

In computer science, the clique problem is the computational problem of finding cliques (subsets of vertices, all adjacent to each other, also called complete subgraphs) in a graph. It has several different formulations depending on which cliques, and what information about the cliques, should be found. Common formulations of the clique problem include finding a maximum clique (a clique with the largest possible number of vertices), finding a maximum weight clique in a weighted graph, listing all maximal cliques (cliques that cannot be enlarged), and solving the decision problem of testing whether a graph contains a clique larger than a given size.

The clique problem arises in the following real-world setting. Consider a social network, where the graph's vertices represent people, and the graph's edges represent mutual acquaintance. Then a clique represents a subset of people who all know each other, and algorithms for finding cliques can be used to discover these groups of mutual friends. Along with its applications in social networks, the clique problem also has many applications in bioinformatics, and computational chemistry. (Full article...)
Image 9
Graph of the equation $y = 1/ x$ . Here, $e$ is the unique number larger than 1 that makes the shaded area under the curve equal to 1.

The number e is a mathematical constant approximately equal to 2.71828 that is the base of the natural logarithm and exponential function. It is sometimes called Euler's number, after the Swiss mathematician Leonhard Euler, though this can invite confusion with Euler numbers, or with Euler's constant, a different constant typically denoted $\gamma$ . Alternatively, $e$ can be called Napier's constant after John Napier. The Swiss mathematician Jacob Bernoulli discovered the constant while studying compound interest.

The number $e$ is of great importance in mathematics, alongside 0, 1, π, and $i$ . All five appear in one formulation of Euler's identity $e^{i\pi }+1=0$ and play important and recurring roles across mathematics. Like the constant $π$ , $e$ is irrational, meaning that it cannot be represented as a ratio of integers, and moreover it is transcendental, meaning that it is not a root of any non-zero polynomial with rational coefficients. To 30 decimal places, the value of $e$ is: (Full article...)
Image 10
Three of the ordinary lines in a 4 × 4 grid of points

The Sylvester–Gallai theorem in geometry states that every finite set of points in the Euclidean plane has a line that passes through exactly two of the points or a line that passes through all of them. It is named after James Joseph Sylvester, who posed it as a problem in 1893, and Tibor Gallai, who published one of the first proofs of this theorem in 1944.

A line that contains exactly two of a set of points is known as an ordinary line. Another way of stating the theorem is that every finite set of points that is not collinear has an ordinary line. According to a strengthening of the theorem, every finite point set (not all on one line) has at least a linear number of ordinary lines. An algorithm can find an ordinary line in a set of $n$ points in time $O(n\log n)$ . (Full article...)
Image 11

An example of non‑periodicity due to another orientation of one tile out of an infinite number of identical tiles

A tessellation or tiling is the covering of a surface, often a plane, using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellation can be generalized to higher dimensions and a variety of geometries.

A periodic tiling has a repeating pattern. Some special kinds include regular tilings with regular polygonal tiles all of the same shape, and semiregular tilings with regular tiles of more than one shape and with every corner identically arranged. The patterns formed by periodic tilings can be categorized into 17 wallpaper groups. A tiling that lacks a repeating pattern is called "non-periodic". An aperiodic tiling uses a small set of tile shapes that cannot form a repeating pattern (an aperiodic set of prototiles). A tessellation of space, also known as a space filling or honeycomb, can be defined in the geometry of higher dimensions. (Full article...)
Image 12
In this graph, an even number of vertices (the four vertices numbered 2, 4, 5, and 6) have odd degrees. The sum of degrees of all six vertices is 2 + 3 + 2 + 3 + 3 + 1 = 14, twice the number of edges.

In graph theory, the handshaking lemma is the statement that, in every finite undirected graph, the number of vertices that touch an odd number of edges is even. For example, if there is a party of people who shake hands, the number of people who shake an odd number of other people's hands is even. The handshaking lemma is a consequence of the degree sum formula, also sometimes called the handshaking lemma, according to which the sum of the degrees (the numbers of times each vertex is touched) equals twice the number of edges in the graph. Both results were proven by Leonhard Euler (1736) in his famous paper on the Seven Bridges of Königsberg that began the study of graph theory.

Beyond the Seven Bridges of Königsberg Problem, which subsequently formalized Eulerian Tours, other applications of the degree sum formula include proofs of certain combinatorial structures. For example, in the proofs of Sperner's lemma and the mountain climbing problem the geometric properties of the formula commonly arise. The complexity class PPA encapsulates the difficulty of finding a second odd vertex, given one such vertex in a large implicitly-defined graph. (Full article...)

Вы знали(сгенерировано автоматически)–загрузить новую партию

... что сложенный бумажный фонарь показывает, что некоторые математические определения площади поверхности неверны?
... что математик Дэниел Ларсен был самым молодым участником кроссворда New York Times ?
... что более 60 научных работ, написанных математиком Полом Эрдёшем, были опубликованы посмертно ?
... что владелец Мэтью Бенхэм повлиял на футбольный клуб «Брентфорд» в Великобритании и футбольный клуб «Мидтъюлланд» в Дании, заставив их использовать математическое моделирование для привлечения недооцененных футболистов?
... что «Катехумен» , христианский шутер от первого лица , финансировался только после бойни в школе «Колумбайн» ?
... что музыку математической рок- группы Jyocho попеременно описывают как «сродни безумию» или «созерцательности и меланхолии»?
... что жители Мадагаскара выполняют алгебраические действия с семенами деревьев, чтобы предсказать будущее ?
... что «Математический миф» призывает американские средние школы прекратить изучение углубленной алгебры?

редактировать

Знаете ли вы больше –просмотреть различные записи

Вы знали...

... что клеточный автомат «Жизнь без смерти» , математическая модель формирования паттернов , является вариантом игры «Жизнь» Конвея , в которой клетки, однажды вызванные к жизни, никогда не умирают?
... что можно перечислить все положительные рациональные числа без повторений, обходя в ширину дерево Колкина–Уилфа ?
... что гипотеза Хадвигера подразумевает, что внешняя поверхность любого трехмерного выпуклого тела может быть освещена всего восемью источниками света, но лучшей доказанной границей является то, что достаточно 16 источников света?
... что справедливая раскраска графа , в которой количество вершин каждого цвета максимально равно, может потребовать гораздо больше цветов, чем раскраска графа без этого ограничения?
... что независимо от того, насколько предвзятой является используемая монета , подбрасывание монеты для определения наличия или отсутствия каждого ребра в счетно бесконечном графе всегда даст один и тот же граф , граф Радо ?
...возможно ли сложить одинаковые костяшки домино на краю стола так, чтобы получился произвольно большой выступ?
...что в алгоритме Флойда для обнаружения цикла черепаха и заяц движутся с очень разной скоростью, но всегда финишируют в одном и том же месте?

редактировать

Избранная статья –показать еще

Алан Матисон Тьюринг , OBE (23 июня 1912 — 7 июня 1954) — английский математик , логик и криптограф .

Тьюринг часто считается отцом современной компьютерной науки . Тьюринг обеспечил влиятельную формализацию концепции алгоритма и вычислений с помощью машины Тьюринга , сформулировав ныне широко принятую «тьюринговскую» версию тезиса Чёрча-Тьюринга , а именно, что любая практическая вычислительная модель имеет либо эквивалент, либо подмножество возможностей машины Тьюринга. С тестом Тьюринга он внес значительный и характерно провокационный вклад в дебаты относительно искусственного интеллекта : можно ли когда-нибудь сказать, что машина обладает сознанием и может мыслить . Позже он работал в Национальной физической лаборатории , создав один из первых проектов компьютера с хранимой программой, хотя он так и не был фактически построен. В 1947 году он переехал в Манчестерский университет , чтобы работать, в основном над программным обеспечением, над Manchester Mark I, который затем стал одним из первых в мире настоящих компьютеров.

Во время Второй мировой войны Тьюринг работал в Блетчли-Парке , британском центре по взлому кодов , и некоторое время возглавлял Hut 8 , секцию, ответственную за криптоанализ немецкого флота. Он разработал ряд методов взлома немецких шифров, включая метод « бомбы» , электромеханической машины, которая могла находить настройки для машины «Энигма» . ( Полная статья... )

редактировать

Подкатегории

Полное дерево категорий. Выберите [►], чтобы просмотреть подкатегории.

Математика

Математика по странам

Области математики

Списки, связанные с математикой

Математики

Работы по математике

Математика и искусство

Математические системы классификации

Математические концепции

Математические константы

Математика и культура

Эпонимы в математике

Математические примеры

Статьи о многогранниках класса FL

История математики

Математическая нотация

Математические науки профессии

Очерки математики и логики

Философия математики

Математические проекты

Математические доказательства

Псевдоматематика

Установить индекс статей по математике

Математическая терминология

Математические теоремы

Математические инструменты

Математические заготовок

редактировать

Темы по математике

редактировать

Индекс статей по математике

редактировать

Связанные порталы

редактировать

WikiProjects

Mathematics WikiProject — центр редактирования в Википедии, связанного с математикой. Присоединяйтесь к обсуждению на странице обсуждения проекта .

В других проектах Викимедиа

Более подробную информацию по этой теме можно найти в следующих родственных проектах Фонда Викимедиа :

Бесплатный репозиторий Commons
Wikibooks
Бесплатные учебники и руководства
Wikidata
Бесплатная база знаний
Wikinews
Новости свободного контента
Wikiquote
Коллекция цитат
Wikisource
Библиотека свободного контента
Wikiversity
Бесплатные инструменты обучения
Викисловарь
и тезаурус

Больше порталов

Откройте для себя Википедию с помощью порталов